Adding and Subtracting Mixed Numbers with Different
Denominators
We have previously shown that, when we add (or subtract)
fractions with different denominators, we must first change the
unlike fractions to equivalent like fractions. The same applies
to adding (or subtracting) mixed numbers that have different
denominators.
EXAMPLE 1
Find the sum of 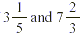 . .
Solution
The denominators have no common factor other than 1. Therefore
the least common denominator is the product of 5 and 3, or 15.
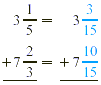
Now we use the rule for adding mixed numbers that have the
same denominator.

The sum of 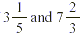 is is 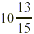 . .
Subtracting mixed numbers that have different denominators is
similar to adding them.
EXAMPLE 2
Subtract  . .
Solution
As usual, we use the LCD (which is 100) to find equivalent
fractions. Then we subtract the equivalent mixed numbers with the
same denominator. Again, let’s set up the problem
vertically.
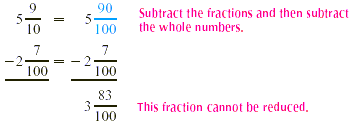
Therefore the answer is 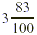 . .
EXAMPLE 3
Find the sum of 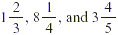 . .
Solution
Set up the problem vertically and use the LCD, which is 60.
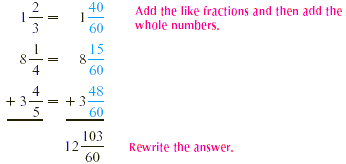
Since 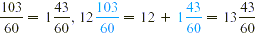 . .
EXAMPLE 4
Find the missing dimension.
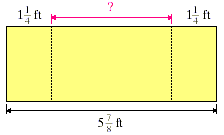
Solution
The total length of the figure is 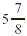 feet. To find the missing dimension, we
need to add feet. To find the missing dimension, we
need to add  feet and feet and  feet and then subtract this sum from feet and then subtract this sum from 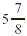 . .
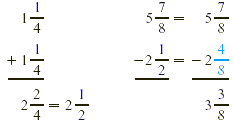
The missing dimension is 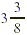 feet. We can check this answer by adding feet. We can check this answer by adding  , , 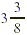 , and , and  , getting , getting 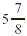 . .
|