Using Slope-Intercept Form for Graphing
In the slope-intercept form, a point on the line (the y-intercept) and the
slope are readily available. To graph a line, we can start at the y-intercept
and count off the rise and run to get a second point on the line.
Example 1
Using slope and y-intercept to graph
Graph the line 2x - 3y = -3.
Solution
First write the equation in slope-intercept form:
| 2x - 3y |
= -3 |
| -3y |
= -2x - 3 |
| y |
 |
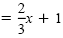The slope is
 ,
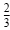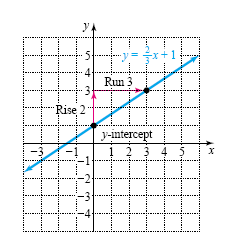
and the y-intercept is (0, 1). Start at (0, 1) on the y-axis, then rise 2 and
run 3 to locate a second point on the line. Because there is only one line
containing any two given points, these two points determine the line. See the
figure below.
Methods for Graphing a Linear Equation
The three methods used for graphing linear equations are summarized as
follows.
Methods for Graphing a Linear Equation
1. Arbitrarily select some points that satisfy the equation, and draw a line
through them.
2. Find the x- and y-intercepts (provided that they are not the origin), and
draw a line through them.
3. Start at the y-intercept and use the slope to locate a second point, then
draw a line through the two points.
|
