Multiplying and Dividing Rational Expressions
Multiplying rational expressions is similar to multiplying fractions in
arithmetic.
Procedure
To Multiply Rational Expressions
Step 1 Factor the numerators and denominators.
Step 2 Cancel all pairs of factors common to the numerators and
denominators.
Step 3 Multiply the numerators, and then multiply the denominators.
Example 1
Simplify:
|
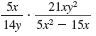
Solution
Step 1 Factor.
Step 2 Cancel common factors.
Step 3 Multiply.
|
 |
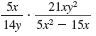
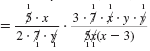
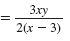The result is .
Note
In your final answer, you may leave the
parentheses. Or, you may use the
Distributive Property to remove them. So,
may be written
as
 .
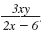Dividing rational expressions is similar to dividing fractions in arithmetic.
Procedure
To Divide Rational Expressions
Step 1 Invert the second rational expression and change
÷ to ·.
Step 2 Multiply.
Example 2
Simplify:
|
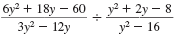
Solution
Step 1 Invert the second rational
expression and change ÷ to
·.
Step 2 Multiply.
Factor.
Cancel.
Simplify. |
|
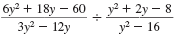
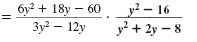
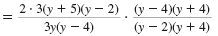
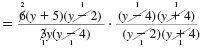
Thus, the result is .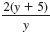
Note:
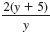can be written as
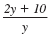
Some rational expressions contain both multiplication and division. To
simplify these expressions, we can begin by changing division to
multiplication.
Example 3
Simplify:
|
Solution
First, convert the division to
multiplication.
Factor.
Cancel pairs of common factors.
Multiply.
|
 |
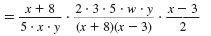
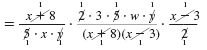
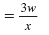The result is
 .
|
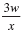