Writing Linear Equations in Slope-Intercept Form
Objective Learn the slope-intercept form of
the equation defining a line.
The slope-intercept form is an extremely useful special case
of the point-slope form of the equation defining a straight line.
It is written as y = mx + b, where m is the slope and b is the y-intercept,
the point where the line intersects the y-axis. The x- and y-intercepts
of a line are the points where the line intersects the x- and y-axes,
respectively.
First, we will try to answer the question “How can we
determine the x- and y-intercepts of a line that is given in the
form of an equation?”
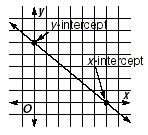
Then we will learn how to find the intercepts algebraically by
solving linear equations. This can be done whether the equations
are in standard form or in point-slope form.
Example 1
Find the y-intercept of the graph of 2x + 3y = 12.
Solution
This equation is in standard form. The y-intercept is the
intersection of the line and the y-axis, so it is a point that is
both on the line and on the y-axis. It satisfies the following
equations.
| 2x + 3y |
= 12 |
Equation of the line |
| x |
= 0 |
Equation of the y-axis |
To find the y-intercept, let x = 0 in the equation of the
line.
| 2x + 3y |
= 12 |
|
| 2(0) + 3y |
= 12 |
Let x = 0. |
| 3y |
= 12 |
|
| y |
= 4 |
Divide each side by 3. |
The y-intercept of this line is 4, so the line crosses the
y-axis at (0, 4).
Example 2
Find the y-intercept of the graph of 2( y - 1) = 5( x + 2).
Solution
This equation is in point-slope form. As in the case of
standard form, let x = 0 and solve for y.
| 2( y - 1) |
= 5( x + 2) |
|
| 2( y - 1) |
= 5( 0 + 2) |
Let x = 0. |
| 2y - 2 |
= 10 |
Distributive Property |
| 2y |
= 12 |
Add 2 to each side. |
| y |
= 6 |
Divide each side by 2. |
So, the y-intercept is 6 and the line crosses the y-axis at
(0, 6).
To find the x-intercept, let y = 0 in the equation, since the
x-axis is given by the equation y = 0. Practice finding the x and
y-intercepts of the graphs of equations.
|