Solving Equations with One Radical Term
Example
Solve for y:
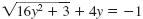
| Solution |
 |
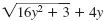= -1 |
| Step 1 Isolate the radical term.
Subtract 4y from both sides. |
 |
= -4y - 1 |
| Step 2 Apply the Principle of Powers.
Square both sides. |
 |
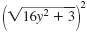= (-4y - 1)2 |
| Step 3 Solve the resulting equation.
Write the right side as a product.
FOIL the right side.
Subtract 16y2 from both sides.
Subtract 1 from both sides.
|
16y2 + 3
16y2 + 3
3
2 |
= (-4y - 1)(-4y - 1)
= 16y2 + 8y + 1
= 8y + 1
= 8y |
| Divide both sides by 8 and reduce. |
 |
= y |
| Step 4 Check the solution. |
|
|
The result
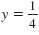 is an extraneous solution.
So, the equa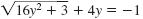tion
 has no solution.
|
